Transmitted Intensity and Linear Attenuation Coefficient
For a narrow beam of mono-energetic photons, the change in x-ray beam intensity at some distance in a material can be expressed in the form of an equation as:
| Where: | dI | = | the change in intensity |
| I | = | the initial intensity | |
| n | = | the number of atoms/cm3 | |
| = | a proportionality constant that reflects the total probability of a photon being scattered or absorbed | ||
| dx | = | the incremental thickness of material traversed |
When this equation is integrated, it becomes:
The number of atoms/cm3 (n) and the proportionality constant () are usually combined to yield the linear attenuation coefficient (m). Therefore the equation becomes:
| Where: | I | = | the intensity of photons transmitted across some distance x |
| I0 | = | the initial intensity of photons | |
| µ | = | the linear attenuation coefficient | |
| x | = | distance traveled |
The Linear Attenuation Coefficient (µ)
The linear attenuation coefficient (µ) describes the fraction of a beam of x-rays or gamma rays that is absorbed or scattered per unit thickness of the absorber. This value basically accounts for the number of atoms in a cubic cm volume of material and the probability of a photon being scattered or absorbed from the nucleus or an electron of one of these atoms.
Using the transmitted intensity equation above, linear attenuation coefficients can be used to make a number of calculations. These include:
- the intensity of the x-ray beam transmitted through a material when the incident x-ray intensity, the material and the material thickness are known.
- the intensity of the incident x-ray beam when the transmitted x-ray intensity, material, and material thickness are known.
- the thickness of the material when the incident and transmitted intensity, and the material are known.
- the material itself can be determined from the value of µ when the incident and transmitted intensity, and the material thickness are known.
Linear attenuation coefficients can sometimes be found in the literature. However, it is often easier to locate attenuation data in terms of the mass attenuation coefficient. Tables and graphs of the mass attenuation coefficients for all of the elements Z = 1 to 92, and for compounds and mixtures of radiological interest are available at the National Institute for Standards and Technology website. The tables on the NIST website cover energies of photons (x-ray, gamma ray, bremsstrahlung) from 1 keV to 20 MeV. A mass attenuation coefficient can easily be converted to a linear attenuation coefficient as discussed below.
Mass Attenuation Coefficient and Conversion to Linear Attenuation Coefficient
Since a linear attenuation coefficient is dependent on the density of a material, the mass attenuation coefficient is often reported for convenience. Consider water for example. The linear attenuation for water vapor is much lower than it is for ice because the molecules are more spread out in vapor so the chance of a photon encounter with a water particle is less. Normalizing m by dividing it by the density of the element or compound will produce a value that is constant for a particular element or compound. This constant is known as the mass attenuation coefficient and has units of cm2/gm.
To convert a mass attenuation coefficient to a linear attenuation coefficient (μ), simply multiply it by the density (ρ) of the material.
Use of Linear Attenuation Coefficients
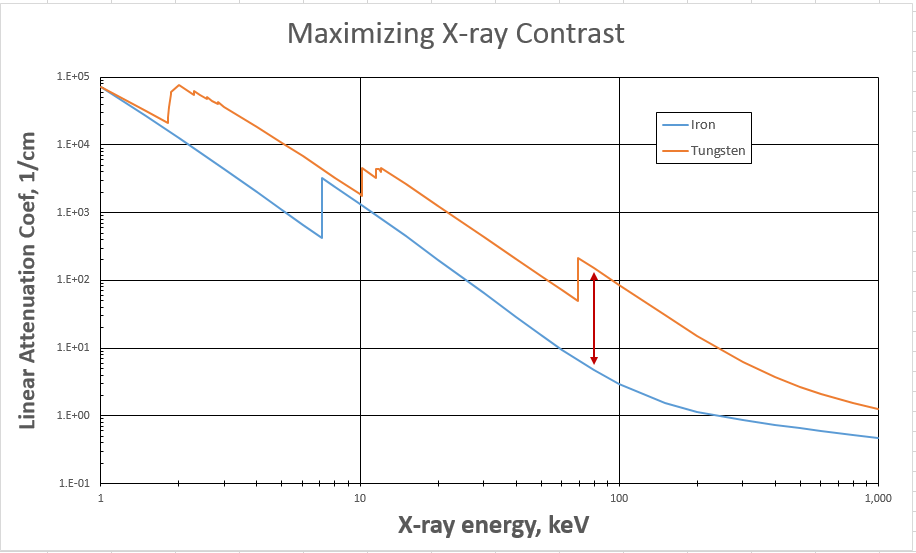
One use of linear attenuation coefficients is for selecting a radiation energy that will produce the most contrast between particular materials in a radiograph. Say, for example, that it is necessary to detect tungsten inclusions in iron. It can be seen from the graphs of linear attenuation coefficients versus radiation energy, that the maximum separation between the tungsten and iron curves occurs at around 100keV. At this energy the difference in attenuation between the two materials is the greatest so the radiographic contrast will be maximized.