Sound Propagation in Elastic Materials

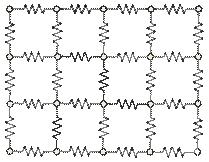In the previous pages, it was pointed out that sound waves propagate due to the vibrations or oscillatory motions of particles within a material. An ultrasonic wave may be visualized as an infinite number of oscillating masses or particles connected by means of elastic springs. Each individual particle is influenced by the motion of its nearest neighbor and both inertial and elastic restoring forces act upon each particle.

A mass on a spring has a single resonant frequency determined by its spring constant k and its mass m. The spring constant is the restoring force of a spring per unit of length. Within the elastic limit of any material, there is a linear relationship between the displacement of a particle and the force attempting to restore the particle to its equilibrium position. This linear dependency is described by Hooke's Law.

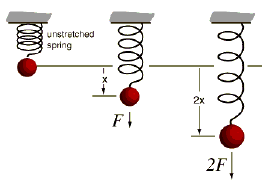In terms of the spring model, Hooke's Law says that the restoring force due to a spring is proportional to the length that the spring is stretched, and acts in the opposite direction. Mathematically, Hooke's Law is written as F =-kx, where F is the force, k is the spring constant, and x is the amount of particle displacement. Hooke's law is represented graphically it the right. Please note that the spring is applying a force to the particle that is equal and opposite to the force pulling down on the particle.

The Speed of Sound

Hooke's Law, when used along with Newton's Second Law, can explain a few things about the speed of sound. The speed of sound within a material is a function of the properties of the material and is independent of the amplitude of the sound wave. Newton's Second Law says that the force applied to a particle will be balanced by the particle's mass and the acceleration of the the particle. Mathematically, Newton's Second Law is written as F = ma. Hooke's Law then says that this force will be balanced by a force in the opposite direction that is dependent on the amount of displacement and the spring constant (F = -kx). Therefore, since the applied force and the restoring force are equal, ma = -kx can be written. The negative sign indicates that the force is in the opposite direction.

Since the mass m and the spring constant k are constants for any given material, it can be seen that the acceleration a and the displacement x are the only variables. It can also be seen that they are directly proportional. For instance, if the displacement of the particle increases, so does its acceleration. It turns out that the time that it takes a particle to move and return to its equilibrium position is independent of the force applied. So, within a given material, sound always travels at the same speed no matter how much force is applied when other variables, such as temperature, are held constant.

What properties of material affect its speed of sound?

Of course, sound does travel at different speeds in different materials. This is because the mass of the atomic particles and the spring constants are different for different materials. The mass of the particles is related to the density of the material, and the spring constant is related to the elastic constants of a material. The general relationship between the speed of sound in a solid and its density and elastic constants is given by the following equation:

$$V=\sqrt{\frac{C_{ij}}{\rho}}$$

Where V is the speed of sound, C_ij is the elastic constant, and ρ is the material density. This equation may take a number of different forms depending on the type of wave (longitudinal or shear) and which of the elastic constants that are used. The typical elastic constants of a materials include:

• Young's Modulus, E: a proportionality constant between uniaxial stress and strain.
• Poisson's Ratio, ν: the ratio of radial strain to axial strain
• Bulk modulus, K: a measure of the incompressibility of a body subjected to hydrostatic pressure.
• Shear Modulus, G: also called rigidity, a measure of a substance's resistance to shear.
• Lame's Constants, λ and μ: material constants that are derived from Young's Modulus and Poisson's Ratio.

When calculating the velocity of a longitudinal wave, Young's Modulus and Poisson's Ratio are commonly used. When calculating the velocity of a shear wave, the shear modulus is used. It is often most convenient to make the calculations using Lame's Constants, which are derived from Young's Modulus and Poisson's Ratio.

It must also be mentioned that the subscript ij attached to C in the above equation is used to indicate the directionality of the elastic constants with respect to the wave type and direction of wave travel. In isotropic materials, the elastic constants are the same for all directions within the material. However, most materials are anisotropic and the elastic constants differ with each direction. For example, in a piece of rolled aluminum plate, the grains are elongated in one direction and compressed in the others and the elastic constants for the longitudinal direction are different than those for the transverse or short transverse directions.

Examples of approximate compressional sound velocities in materials are:

• Aluminum - 0.632 cm/microsecond
• 1020 steel - 0.589 cm/microsecond
• Cast iron - 0.480 cm/microsecond

Examples of approximate shear sound velocities in materials are:

• Aluminum - 0.313 cm/microsecond
• 1020 steel - 0.324 cm/microsecond
• Cast iron - 0.240 cm/microsecond

When comparing compressional and shear velocities, it can be noted that shear velocity is approximately one half that of compressional velocity. The sound velocities for a variety of materials can be found in the ultrasonic properties tables in the general resources section of this site.