Impedance

After reading this section you will be able to do the following:

  • Define what impednace is
  • Calculate impedance

Electrical Impedance (Z), is the total opposition that a circuit presents to alternating current. Impedance is measured in ohms and may include resistance (R), inductive reactance (XL), and capacitive reactance (XC). However, the total impedance is not simply the algebraic sum of the resistance, inductive reactance, and capacitive reactance. Since the inductive reactance and capacitive reactance are 90o out of phase with the resistance and, therefore, their maximum values occur at different times, vector addition must be used to calculate impedance. Additionally, how components like resistors, inductors, and capacitors, are arranged in a circuit also play a role in what the total impedance of the circuit is. More discussion on circuits and circuit analysis is provided later in this module. 

Example: Impedance of a Circuit

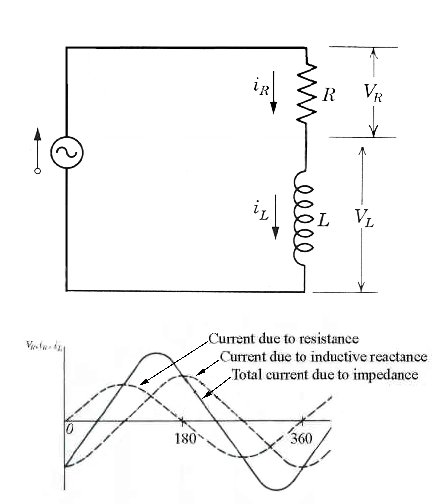
In the image below, a circuit diagram is shown that represents an eddy current inspection system. The eddy current probe is a coil of wire so it contains resistance and inductive reactance when driven by alternating current. The capacitive reactance can be dropped as most eddy current probes have little capacitive reactance. The solid line in the graph below shows the circuit's total current, which is affected by the total impedance of the circuit. The two dashed lines represent the portion of the current that is affected by the resistance and the inductive reactance components individually. It can be seen that the resistance and the inductive reactance lines are 90o out of phase, so when combined to produce the impedance line, the phase shift is somewhere between zero and 90o. The phase shift is always relative to the resistance line since the resistance line is always in-phase with the voltage. If more resistance than inductive reactance is present in the circuit, the impedance line will move toward the resistance line and the phase shift will decrease. If more inductive reactance is present in the circuit, the impedance line will shift toward the inductive reactance line and the phase shift will increase. This concept is discussed more in the Circuits and Phase page.

A typical eddy current coil has a resistor and an inductor. The phase of the current is effected by the presence of both of these components.

Vector Analysis

The relationship between impedance and its individual components (resistance and inductive reactance) can be represented using a vector as shown below. A vector is a representation of a quantity that has both a magnitude and a direction, which in this case is represented by a phase angle (see the figure below).

The amplitude of the resistance component is shown by a vector along the x-axis and the amplitude of the inductive reactance is shown by a vector along the y-axis. The amplitude of the the impedance is shown by a vector that stretches from zero to a point that represents both the resistance value in the x-direction and the inductive reactance in the y-direction. Eddy current instruments with impedance plane displays present information in this format.

The angle between the resistance and the inductive reactance is the phase angle.

The impedance in a circuit with resistance and inductive reactance can be calculated using the following equation. If capacitive reactance was present in the circuit, its value would be added to the inductance term before squaring.

Z = ( X L 2 + R 2 ) Z=\sqrt{(X^{2}_{L}+R^{2})}

The phase angle of the circuit can also be calculated using some trigonometry. The phase angle is equal to the ratio between the inductance and the resistance in the circuit. With the probes and circuits used in nondestructive testing, capacitance can usually be ignored so only inductive reactance needs to be accounted for in the calculation. The phase angle can be calculated using the equation below. If capacitive reactance was present in the circuit, its value would simply be subtracted from the inductive reactance term.

tan ⁡ ϕ = X L R \tan\phi=\frac{X_{L}}{R}

ϕ = arctan ⁡ X L R \phi= \arctan \frac{X_{L}}{R}

The applet below can be used to see how the variables in the above equation are related on the the vector diagram (or the impedance plane display). Values can be entered into the dialog boxes or the arrow head on the vector diagram can be dragged to a point representing the desired values. Note that the capacitive reactance term has been included in the applet but as mentioned before, in eddy current testing this value is small and can be ignored.

Review:

  1. Impedance is is the total opposition that a circuit presents to alternating current.
  2. Vector addition is used to calculate impedance.